Cluster World

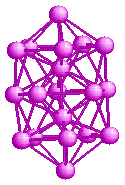
Structure of strontium clusters

Micro world is governed by atoms and molecules, and the macro world consists of materials which contain a mind-boggling number of atoms or molecules. For atoms and molecules we study chemistry - we talk of chemical bonds, we talk of electronic orbitals, we talk of reactivity of a molecule. In the macro-world we talk of properties like electrical resistance, thermal conductivity, stiffness. We see distinct phases of materials - liquid, solid and gas.

The bulk consists of atoms and molecules, so the properties of the atoms and molecules should decide the properties of the bulk. This is a challenging problem for scientists and only a little progress has been made in this direction. One would like to see how things change as one goes from atoms and molecules to small assemblies of them, to large assemblies, to larger assemblies, and finally to the bulk. One would like to see how various atoms, which do not appear very different individually, work together to form metals, insulators and semiconductors.

The study of clusters is aimed at looking at this transition from the atomic state to the bulk.

How are small clusters made?

The most popular technique to produce atomic clusters is called the gas aggregation technique. Here, a sample of the material whose clusters are needed is heated in a furnace to such high temperatures that it evaporates. In this vapour phase the material separates out into individual atoms. This gas is mixed with a jet of some inert gas which carries it forward. This inert gas is at low temperatures, and so the atoms of the original material collide these and lose energy. Once they lose energy, they can stick to each other better. 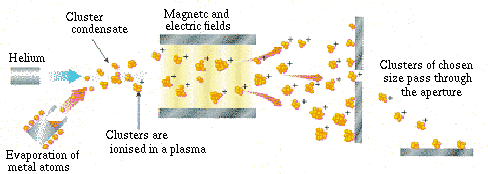So they start forming aggregates. The mass of these resulting clusters is analyzed by a mass spectrometer.

It turns out that in this primeval soup of atoms, clusters of some size are much more abundant than others. These clusters containing certain special number of atoms seem to be much more stable compared to others. These numbers are referred to as magic numbers. In this primeval soup clusters collide with each other and lose atoms. So, only the clusters which are more stable will be found to be abundant. Thus, the magic numbers indicate the stability of certain clusters. The task of a theoretian would be to explain this stability of some clusters, or in other words, explain the magic numbers.

Magic numbers for rare-gases

The magic numbers seen for rare-gas clusters, like Argon, Krypton, Neon are well understood. The magic numbers seen for these are 7, 13, 19, 23, 55. These numbers correspond to nice close-packed structures which can be formed by packing spheres, as shown below.

So, the special stability of rare-gas clusters is believed to be due to this geometric close-packing of atoms in the clusters.

Magic numbers for metal clusters

The clusters of various metals were seen to show magic numbers which are very different from that of rare-gases. Experiments were done on Alkali metals like Lithium, Sodium, Potassium, and other metals like Aluminium, which showed that the clusters are stable when the total number of free electrons in the cluster are 8, 20, 34, 40, 58, .... Notice that here the number of atoms in a cluster is not important - what is important is the total number of free electrons.

This can be understood with the help of what is called the Jellium model. In the Jellium model, one assumes that the outer electrons of the metal atoms are loosly bound so that when a cluster is formed, they can move around everywhere in the clusters, and are not bound to a particular atom. The atoms without their outer electrons are positively charged ions. So, the free electrons "see" a jellium of positive ions in the cluster. The electrons move around in the positive jellium. If one tries to theoretically solve the problem of free electrons in a positive jellium, one indeed observes that the clusters are energetically most stable when the total number of electrons in them are 8, 20, 34, 40, 58 etc! This simple picture can be applied to many metals and works very well.

Experiments on strontium clusters

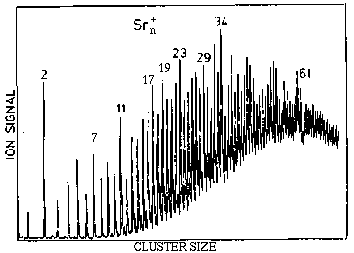C. Brechignac and collaborators performed experiments on Sr clusters. The "abundance spectrum" they obtained is shown in the figure. Although strontium is a metal, it does not show magic numbers similar to the Jellium model. One the other hand, the magic number seen are closer to those for rare-gases. This is strange, because strontium atoms cannot have a weak binding force as in rare-gases - so what is the reason for this kind of magic numbers? Also, one would notice that there is a magic number 11 which is not seen in rare-gases.

Let us have a look at the abundance spectra for Barium and Ytterbium. Surprisingly, Ba and Yb also show magic numbers close to those of rare-gases! So, what is common between these three elements? Their electronic configuration looks like this:

All of these three elements have a ns2 "closed shell" configuration, which means that to add one more electron to the atom, a new shell has to be added. All of these also have the d-states as the immediate next unoccupied states. It appears that this feature may have something to do with the similar kind of magic numbers seen in the clusters of these elements.

Car-Parrinello molecular dynamics simulation

Atoms which are not very light, are generally expected to behave like classical particles. So, it makes sense to do a computer simulation of clusters by letting the atoms evolve via Newton's equations of motion. For this one just needs the knowledge of the initial position and velocities of the atoms, and the forces between them. The calculation of force between two atoms is a non-trivial job because this force has its roots in the electronic structure of the atoms. The electronic structure has to be treated quantum mechanically. This calculation is tedious and can only be done approximately.

The tool one uses to calculate the electronic structure of the cluster is the density functional theory introduced by Walter Kohn. Using this one can calculate the ground state energy of the cluster. M. Parrinello and R. Car came up with an innovative method which combines the density functional calculation for electrons and classical molecular dynamics for atoms, in a single unified formalism. This method has come to be known as the Car-Parrinello molecular dynamics (CPMD).

---Inclusion of d-electrons leads to---->